Circling Geometries #1: the moebius strip
Drawing a line on a Moebius band, one full circle of the pen along the paper returns you to the same place, but on the other side, separated from your starting point by the thickness of the band.

Woman facing a water cannon in Vali Asr Circle, Tehran, 2022
circular movements and spaces, circular and cyclical temporal forms
The filmTrop tôt, trop tard, produced by French filmmakers Danièle Huillet and Jean-Marie Straub in 1982, starts with a camera, mounted on a car driving repeatedly around the Place de la Bastille in Paris. The camera points outwards catching cars passing in other lanes, the buildings encircling the square, and the surrounding cityscape and a wide sky dotted with clouds.
In total, the camera circles the square 14 times.
geometries of change
Geometrien der Versammlung

Members of the left GDR opposition gathered on September 20, 1990 at another Round Table outside of the Palast der Republik (parliament of the GDR) to protest the signing of the German Reunification Treaty. The table was a cable reel from the Kabelwerk Oberspree cable plant, whose staff faced the threat of mass layoffs since the plant had been taken over by the GDR’s Treuhand (trust agency established to re/privatise GDR enterprises post-unification).
In a circle, the starting point of a circumambulation and and its end point appear the same. A two-dimensional circling seemingly returns its circumambulators to the exact same place. The circle’s circumference C describes the distance covered in one such circling. Point B, diametrically opposed to Point A, is at the maximal distance from this starting point A. Completing the circle from B all the way around and back to A, can be perceived as a return.
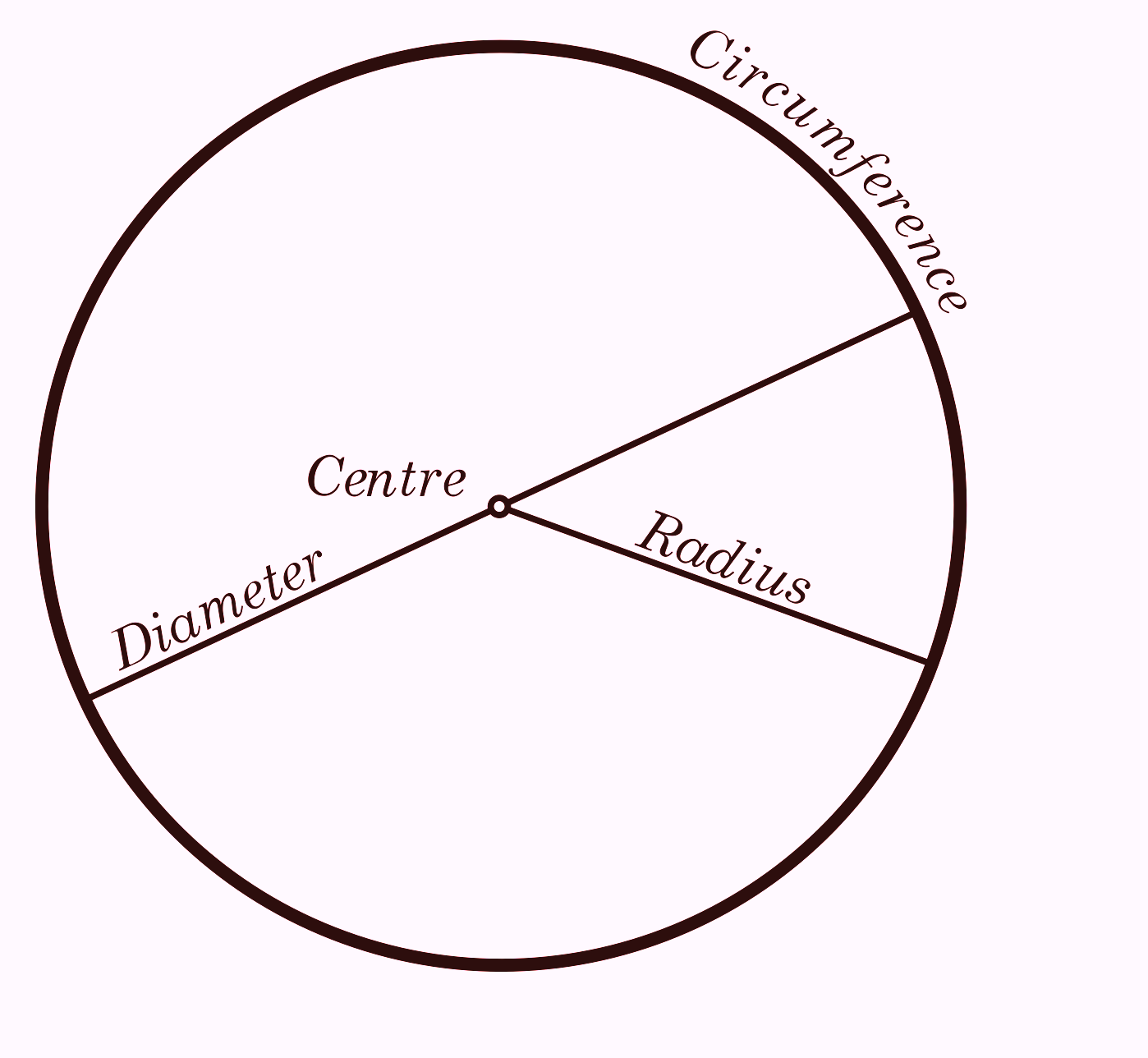
Circling Geometries #2: diameter vs. circumference

Film Still, Trop tôt, trop tard
In the second part of the film Trop tôt, trop tard, the camera is stationary, but turns around itself to scan the full circle of the landscape surrounding it towards the horizon. These shots were filmed in Cairo and the Egyptian countryside. From the circling camera, the narration shoots off tangents into different chronologies of the workers’ and peasants’ revolts that happened at the sites of the filming or spread from there through the width and breadth of the country at different points through its (colonial) history.
Uprising ︎︎︎
︎︎︎Defeat Defeat︎︎︎
︎︎︎Uprising
Infinity is a circle, folded down the middle into a horizontal 8
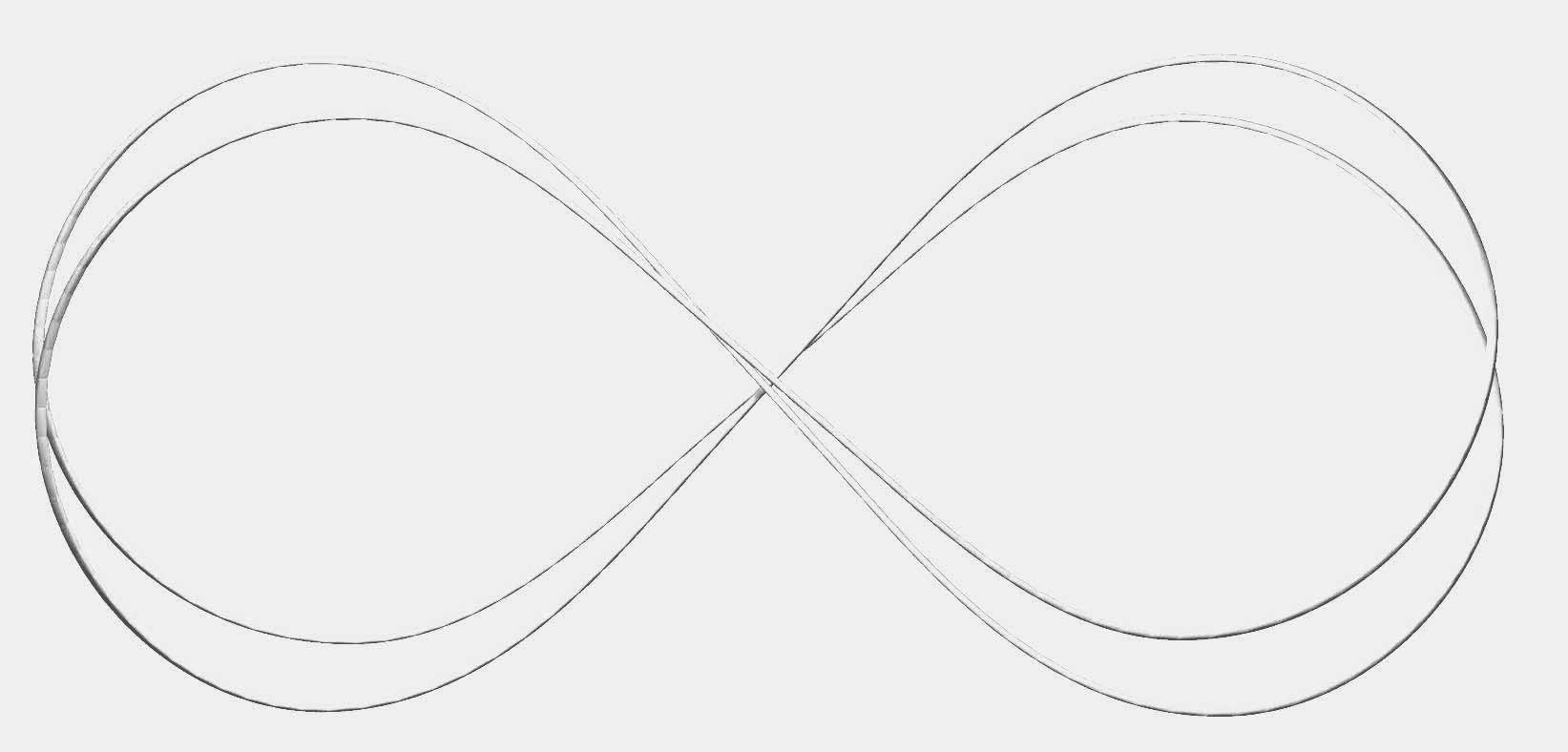
Circling Geometries #3: the infinity sign

Anthropologist Victor Turner and performance theorist Richard Schechner developed a circular – 8-shaped – model of social change: social action and experience during revolution or protest turn into an implicit social process that is then narrativized into drama, from where it provides the implicit rhetorical structure that allows the next event of social action to take its concrete shape.
Language ︎︎︎︎︎︎Experience Experience︎︎︎
︎︎︎Language

In On Speeches, dancer and choreographer Jasmin İhraç performs gestures of spinning and circling over a soundtrack consisting of political speeches by Angela Davis, Cornell West, and Slavoj Zizek.
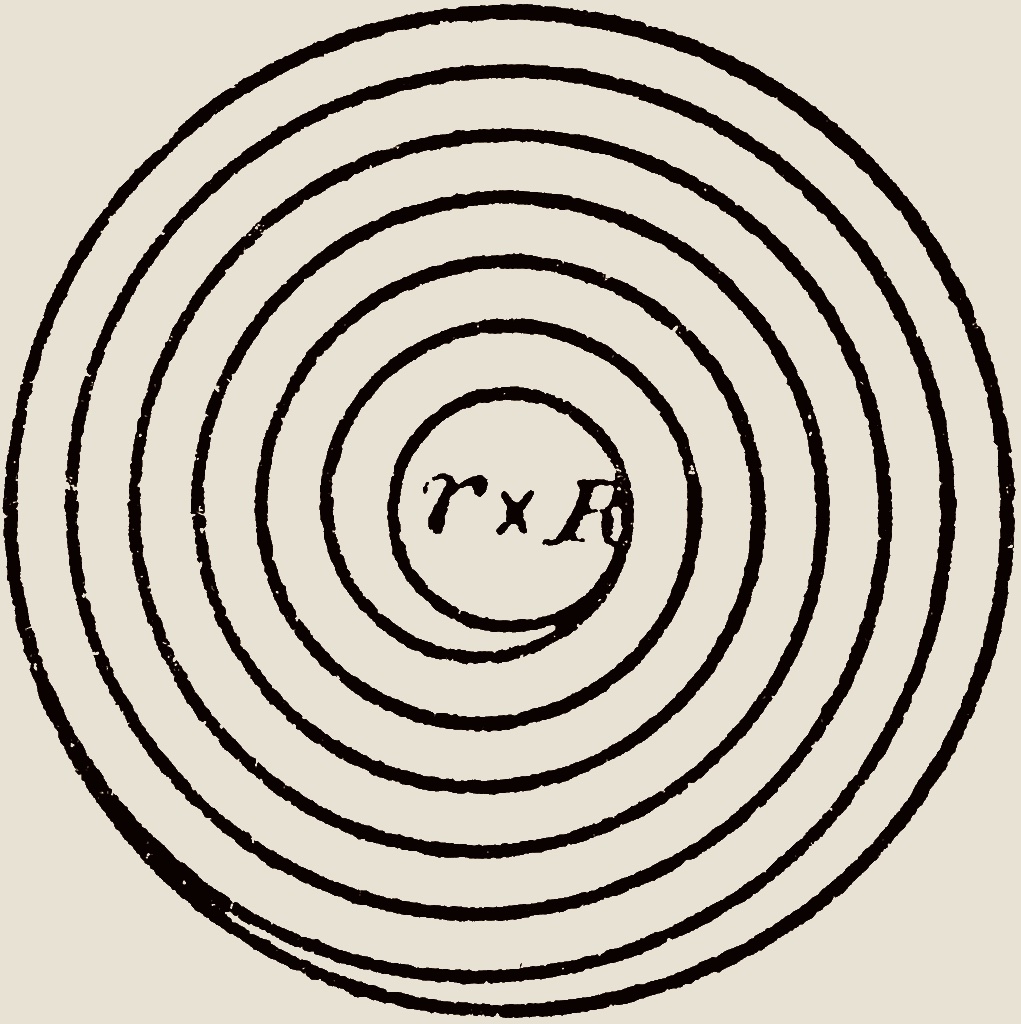
Circling Geometries # 4:
the spiral
twirling, spiralling, spinning around
circling is learning
by losing
control

вре́мя: circular time
Etymologically the Russian word vremya (“time”) comes from the Ancient Slavonic v˴ rtmen (“turning”) and the Ancient Indian root vart – “turning”.
“Time accelerates. The senses are shaken. Hours pass rapidly between one gathering and the next, but then time slows down. The nights are loooong. Time contracts and expands, moved by a sea of people (principally but not only young people). It feels like we’ve been here for years, and it hasn’t been more than three days.”
“Seven key words on the Madrid-Puerta del Sol experience,” 15M, Guillermo Kaejane, 2011

The Mothers of the Plaza de Mayo have been circling one of the main squares of the Argentinian capital Buenos Aires since 1977 in weekly circumambulations to demand the investigation of the disappearance – the assassination – of their children and grandchildren by the military junta which was in power between 1976 and 1983. ︎︎︎
By April 2020, the mothers had circled the square 2194 times
To circle is to keep moving while remaining in place.
technologies of revolution,
technologies of historiography
stop, rewind, fast-forward
collective figures
Isadora Duncan, Revolutionary Etude (1921)
Isadora Duncan (1877-1927) is regarded as a revolutionary of modern dance. Her politics was radical, too. In 1921 she moved to the Soviet Union to take part in the revolution and founded a dance school there, which later failed, due to lack of support from the Soviet state. Until today, her dances are passed down through the generations through direct transmission from dancer to dancer without the use of notation or visual aids.
from body to body
Dance/ ︎︎︎
Slightly altered
Dance
︎︎︎ History
Performance︎︎︎
︎︎︎Body of the
Dancer
Dance Notation
Circling Geometries #5: from circle to spring
In 3D, a circle folds out into a helical spring. A circling is complexified: Seen from the top, a full circle returns you to the same place. Seen from the side, the circling reveals its irreversibility as the distance between the two loops. Change and non-change coexist, what you see is a function of from where you look.
after the end...
“The nation’s [same] backscratchers, those perpetual opportunists, who flourish on the dung of whichever system, are the ones that have made sure they are safe again, that nod along with everything, and come out on top no matter what.”
(East German worker, interviewed in July 1990
(East German worker, interviewed in July 1990
“I would do it again, in exactly the same way. I would wear myself out again, fighting in the exact same wrong way, that is, of course, in the exact same right way, with the exact same non-effect. I would not do anything differently at all. And in the end, I would be a little wiser for it all the same.”
(another East German worker, interviewed in July 1990).
︎︎︎
...before
the beginning.
 Banners, Dresden, 1989
Banners, Dresden, 1989“Aren’t you afraid of a second revolution in the East?”
was a question directed by a TV-interviewer to Carsten Rohwedder, the then head of the Treuhand Agency (the agency tasked with the privatisation of East Germany’s formerly state owned enterprises). The question points to the largely forgotten history of post-1990 protests in East Germany.︎︎︎
History is a helical spring.

Berlin, former Palace of the Republic, July 1991
In a helical spring, a circling remains forever incomplete.
...1989
2011
2013
2022...
The potential energy held by a (ideal) spring is a product of a coefficient – the qualities of the spring, its material, thickness, its diameter – and the length of the displacement from its rest position. To press down a spring requires work, that is, the magnitude of the force times the displacement (in the direction of the force). To keep the spring still, that is, from expanding once more, force is required, constantly.
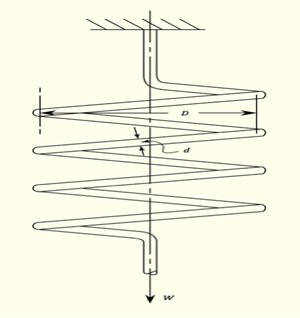
Circling Geometries #6:
the physics of compression.





 Film Still, Trop tôt, trop tard
Film Still, Trop tôt, trop tard

 Simone Forti, Zero, 1975
Simone Forti, Zero, 1975